In our previous blog post, we explored the associative property in general, which states that the way we group numbers in addition or multiplication does not change the final result. In this article, we will focus specifically on the associative property of multiplication. Understanding this property is essential for simplifying calculations and solving multiplication problems efficiently.
What is the Associative Property of Multiplication?
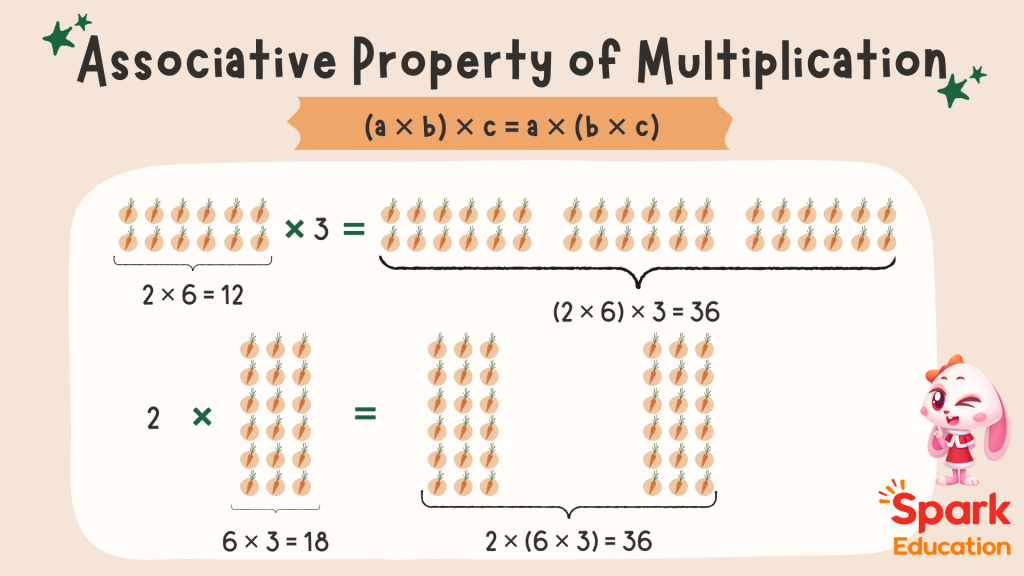
The associative property of multiplication states that when multiplying three or more numbers, the way in which the numbers are grouped does not change the product. In mathematical terms, this can be expressed as:
(a × b) × c = a × (b × c)
This means that whether you multiply the first two numbers together and then multiply by the third number, or you multiply the last two numbers together first, the result will always be the same.
For example:
If we take the numbers 2, 3, and 6:
(2 × 3) × 6 = 6 × 6 = 36
2 × (3 × 6) = 2 × 18 = 36
In both cases, the product is 36. This property allows us to rearrange our calculations to make them easier, especially when working with larger numbers or when doing mental math.
Examples of the Associative Property of Multiplication
To further illustrate the associative property of multiplication, let’s explore a few more examples:
Example 1: Multiply 5, 2, and 6.
(5 × 2) × 6 = 10 × 6 = 60
5 × (2 × 6) = 5 × 12 = 60
Example 2: Multiply 3, 7, and 2.
(3 × 7) × 2 = 21 × 2 = 42
3 × (7 × 2) = 3 × 14 = 42
These examples demonstrate that no matter how we group the numbers, the product remains consistent. This reliability makes the associative property a valuable tool for simplifying multiplication tasks.
Practical Applications of the Associative Property of Multiplication
The associative property can significantly speed up calculations in real-life scenarios. Here are a few ways to apply it:
Breaking Down Complex Problems
When faced with a multiplication problem like 12 × 15 × 4, you can regroup the numbers to make the math simpler. Fo example, instead of calculating directly, you can calculate (15 × 4) × 12 = 60 × 12 = 720, which might be easier.
Mental Math Shortcuts
The associative property is especially useful for mental math. For example, if you need to calculate 8 × 25 × 4, it can be easier to calculate (25 × 4) × 8 = 100 × 8 = 800.
Simplifying Word Problems
Consider a scenario where you need to find the total number of pencils if each student in a class of 20 has 9 packs of 5 pencils each. Instead of calculating 20 × 9 × 5 directly, you could regroup as follows:
(20 × 5) × 9 = 100 × 9 = 900 pencils.
Frequently Asked Questions (FAQ)
Q: What is the associative property of multiplication in simple terms?
A: The associative property of multiplication means that when multiplying three or more numbers, the way you group them doesn’t affect the product.
Q: Can you give an example of the associative property of multiplication?
A: Sure! For example, with the numbers 3, 4, and 5: (3 × 4) × 5 = 12 × 5 = 60 and 3 × (4 × 5) = 3 × 20 = 60.
Q: How is the associative property different from the commutative property?
A: The associative property refers to grouping numbers, while the commutative property refers to changing the order of numbers in multiplication or addition.
Q: Why is the associative property important in math?
A: It allows for flexibility in calculations, making complex multiplication problems easier to solve and understand.
Q: Does the associative property apply to subtraction or division?
A: No, the associative property only applies to addition and multiplication, not to subtraction or division.
By mastering the associative property of multiplication, you can approach math problems with more confidence and efficiency. The next time you encounter a multiplication problem, remember that how you group the numbers can make a big difference in how easily you solve it!
Interested in taking your child’s math skills to the next level? Sign up for a FREE trial class with Spark Math by Spark Education today or try our FREE Online Math Assessment for a detailed report on your child’s math skills! Spark Math is the flagship math course under Spark Education, offering small group classes taught by experienced and engaging real-life teachers. Our program is designed to ignite your child’s passion for learning math, providing a rich array of math resources and an immersive learning experience. Come and see how Spark Math can make a difference in your child’s education!




