In mathematics, factors play a crucial role in solving a variety of problems. By understanding the factors of a number, we can break down its structure and gain insights into its properties. One such number is 630. But what exactly are the factors of 630? How can we find them, and what is their significance?
In this blog, we’ll dive into the factors of 630, explore its prime factorization, and answer some key questions, such as: What is the greatest prime factor of 630? By the end, you’ll have a clear understanding of how to approach factorization problems like this one.
What Are the Factors of 630?
A factor of a number is an integer that divides that number without leaving a remainder. In simpler terms, factors are numbers that can be multiplied together to give the original number.
Let’s take 630 as an example. The factors of 630 are all the integers that can divide 630 evenly. When we calculate the factors of 630, we get the following list: 1, 2, 3, 5, 6, 7, 9, 10, 14, 15, 18, 21, 30, 35, 42, 45, 63, 70, 90, 105, 126, 210, 315, and 630.
To understand this better, consider the factor pairs of 630:
- 1 × 630 = 630
- 2 × 315 = 630
- 3 × 210 = 630
- 5 × 126 = 630
- And so on…
Each of these pairs multiplies to give the original number, which is 630 in this case. Understanding the factor pairs helps us see how the number is constructed from smaller components.
Prime Factorization of 630
Prime factorization is the process of breaking down a number into its smallest prime factors. Prime numbers are numbers that can only be divided by 1 and themselves. To perform prime factorization, we repeatedly divide 630 by prime numbers until we can no longer divide.
Here’s how the prime factorization of 630 works:
Step 1: Start by dividing by the smallest prime number, which is 2.
630 ÷ 2 = 315
Step 2: 315 is not divisible by 2, so move on to the next prime number, which is 3.
315 ÷ 3 = 105
Step 3: 105 is still divisible by 3, so divide again.
105 ÷ 3 = 35
Step 4: Now, divide 35 by the next smallest prime number, which is 5.
35 ÷ 5 = 7
Step 5: The remaining number is 7, which is a prime number and cannot be divided further.
Thus, the prime factorization of 630 is:
630 = 2 × 3 × 3 × 5 × 7
This prime factorization breaks 630 down into its simplest building blocks. Each of these primes is a component of the number, and when multiplied together, they give us the original value of 630.
What Is the Greatest Prime Factor of 630?
Among the prime factors of 630—2, 3, 5, and 7—the greatest prime factor is 7. This is simply the largest prime number that divides 630 evenly. Prime factors are useful for various mathematical applications, including finding the greatest common factor (GCF) or simplifying fractions.
Visualizing the Factors of 630
Sometimes, visual aids can help make the factorization process clearer. One of the best ways to visualize prime factorization is by using a factor tree.
To create a factor tree for 630:
- Start with 630 at the top.
- Divide 630 by the smallest prime factor (2), and place 2 and 315 below it.
- Divide 315 by the next smallest prime factor (3), and place 3 and 105 below it.
- Continue this process, dividing 105 by 3, then 35 by 5, and finally 7 (which is already prime).
Your factor tree will look something like this:
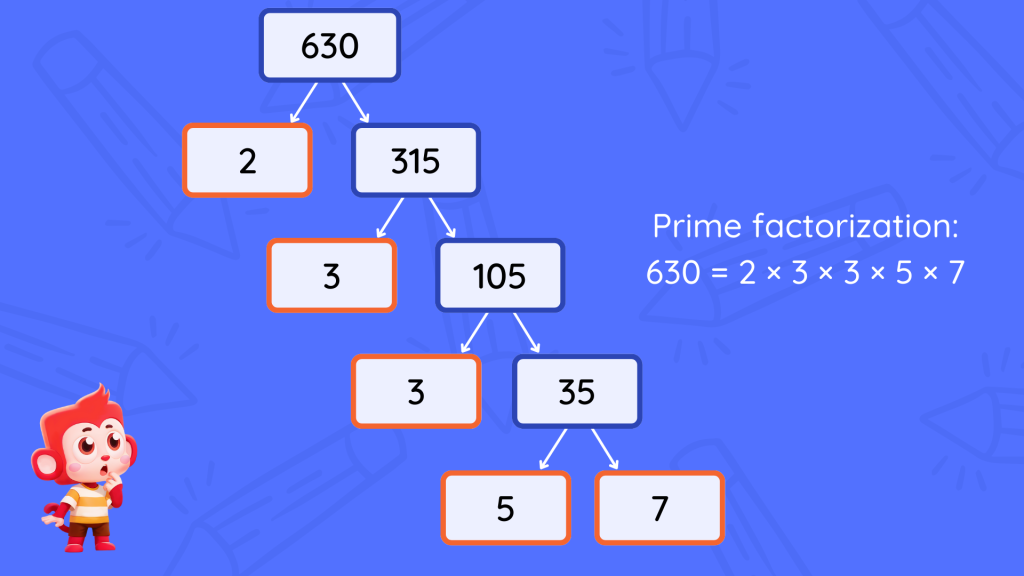
This factor tree shows the breakdown of 630 into its prime factors: 2, 3, 3, 5, and 7. Using visual tools like this can make the factorization process much more intuitive.
Frequently Asked Questions (FAQ)
What is the difference between factors and multiples?
Factors are numbers that divide evenly into another number, while multiples are the result of multiplying a number by an integer. For example, the factors of 630 include 1, 2, and 3, while the multiples of 630 are numbers like 630, 1260, 1890, and so on.
How do you find the factors of 630?
To find the factors of 630, you can start by dividing 630 by smaller numbers to see which divide evenly (i.e., without leaving a remainder). The complete factor list for 630 includes: 1, 2, 3, 5, 6, 7, 9, 10, 14, 15, 18, 21, 30, 35, 42, 45, 63, 70, 90, 105, 126, 210, 315, and 630.
What is the sum of the factors of 630?
To calculate the sum of all factors of 630, you simply add all of its factors together: 1 + 2 + 3 + 5 + 6 + 7 + 9 + 10 + 14 + 15 + 18 + 21 + 30 + 35 + 42 + 45 + 63 + 70 + 90 + 105 + 126 + 210 + 315 + 630 = 1883.
Practice Questions
Now that you have a good understanding of the factors of 630, let’s solidify your knowledge with a few practice problems:
1.What is the greatest common factor (GCF) of 630 and 210?
To find the GCF, you can use the prime factorization method. The prime factorization of 630 is 2 × 3 × 3 × 5 × 7, and the prime factorization of 210 is 2 × 3 × 5 × 7. The GCF is the product of all the common prime factors, which is 210.
2. How many prime factors does 630 have?
The prime factorization of 630 is 2 × 3 × 3 × 5 × 7, so there are 5 prime factors (counting duplicates).
3.Find the least common multiple (LCM) of 630 and 315.
The prime factorization of 630 is 2 × 3 × 3 × 5 × 7, and the prime factorization of 315 is 3 × 3 × 5 × 7. To find the LCM, take the highest power of each prime factor:
LCM = 2 × 3² × 5 × 7 = 1260.
4.Is 630 divisible by 11? To check if a number is divisible by 11, you can apply the rule of alternating sums. For 630, take the alternating sum of its digits: 6 – 3 + 0 = 3. Since 3 is not divisible by 11, 630 is not divisible by 11.
5.What is the smallest prime factor of 630?
The smallest prime factor of 630 is 2, as it divides 630 evenly.
Conclusion
Understanding the factors of a number like 630 gives us insight into its mathematical structure. We’ve explored the full list of factors, performed prime factorization, and found that the greatest prime factor of 630 is 7. Visual tools like factor trees make the factorization process easier to grasp, and solving practice problems helps reinforce these concepts.
By breaking numbers down into their components, we unlock new ways of solving mathematical problems, simplifying calculations, and identifying relationships between different numbers. Whether you’re tackling school assignments or preparing for exams, mastering factorization is a valuable skill that will serve you well in various areas of math.
Interested in taking your child’s math skills to the next level? Sign up for a FREE trial class with Spark Math by Spark Education today or try our FREE Online Math Assessment for a detailed report on your child’s math skills! Spark Math is the flagship math course under Spark Education, offering small group classes taught by experienced and engaging real-life teachers. Our program is designed to ignite your child’s passion for learning math, providing a rich array of math resources and an immersive learning experience. Come and see how Spark Math can make a difference in your child’s education!




