Quick Answer: An obtuse triangle can only have one obtuse angle. This is because the sum of the angles in any triangle must always equal 180 degrees. Since an obtuse angle is greater than 90 degrees, the other two angles must be acute (less than 90 degrees) to ensure the total remains 180 degrees.
Angles are everywhere, from the sharp corners of a book to the open blades of a pair of scissors. But not all angles are created equal, especially when it comes to obtuse angles. These “larger-than-right” angles often leave us with interesting questions, like today’s big question: How many obtuse angles can an obtuse triangle have?
But before we dive into the answer, let’s have a little fun with a geometry-themed joke:
Why did the obtuse angle go to the beach?
Because it was over 90 degrees!
This joke may sound a bit “angle-y,” but it highlights what makes obtuse angles so interesting—they are always larger than a right angle. In this article, we’ll explore what makes an angle obtuse, how many obtuse angles an obtuse triangle can have, and some real-world examples of obtuse angles.
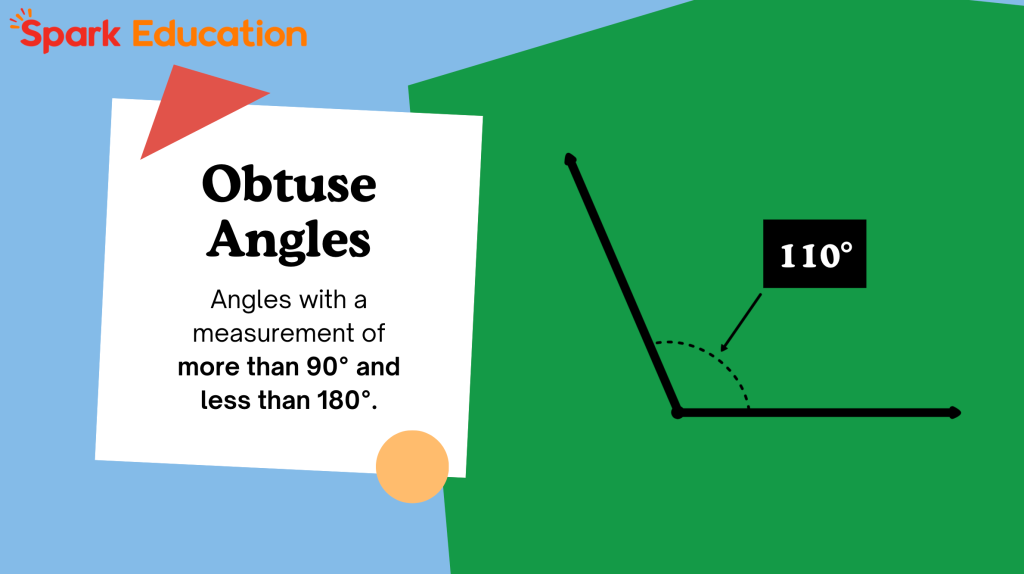
What Is an Obtuse Angle?
In mathematical terms, an obtuse angle is defined as any angle greater than 90 degrees but less than 180 degrees. It’s larger than a right angle, but it doesn’t stretch out to form a straight line (which would be 180 degrees).
To put it simply:
- A right angle is exactly 90 degrees.
- An acute angle is less than 90 degrees.
- An obtuse angle, on the other hand, is anything between 90 and 180 degrees.
In everyday life, obtuse angles are often found in objects that open or spread out beyond a right angle. For example:
- The angle formed when you open a door slightly wider than halfway.
- The angle between the hour and minute hands of a clock at 10:15.
Now that we know what an obtuse angle is, let’s dive into the core of the topic: obtuse triangles.
How Many Obtuse Angles Are in an Obtuse Triangle?
An obtuse triangle is defined as a triangle in which one of the angles is obtuse. Since the total sum of a triangle’s interior angles must always be 180 degrees, an obtuse triangle can only have one obtuse angle. The other two angles must be acute (each less than 90 degrees) to make sure the total adds up correctly.
For example:
- If one angle in the triangle is 120 degrees (an obtuse angle), the remaining two angles must together total 60 degrees. These two would be acute angles, ensuring the overall sum is 180 degrees.
Thus, it’s impossible for a triangle to have more than one obtuse angle.
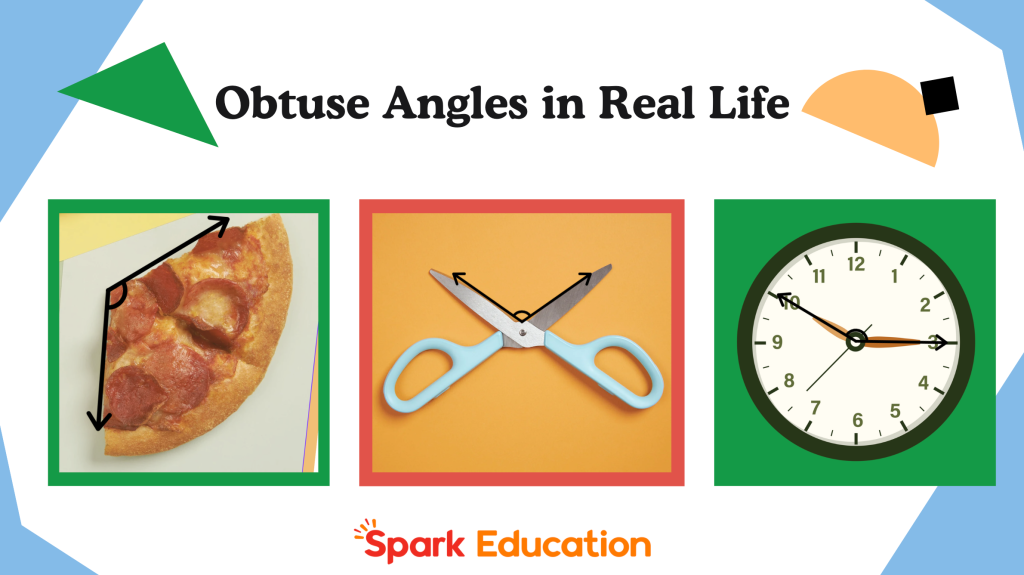
Obtuse Angles in Real Life
Obtuse angles aren’t just limited to geometry textbooks—they appear frequently in the world around us. Here are some fun and relatable examples of obtuse angles in real life:
Pizza Slices: When you slice a pizza, some slices may have angles greater than 90 degrees, especially if the pizza is cut unevenly. These are obtuse angles!
Ramps and Slides: Many ramps and playground slides are designed with obtuse angles to provide a gentle slope that’s fun yet safe for children.
Open Books: When you open a book and lay it flat on a table, the angle between the two open pages is often obtuse.
Scissors: As you open a pair of scissors, the angle between the blades widens to become obtuse.
Clock Hands: At certain times, such as 10:15, the angle between the hour and minute hands forms an obtuse angle.
These everyday objects and scenarios make it easier to spot obtuse angles and understand how they work in both practical and playful settings.
FAQ: Common Questions About Obtuse Angles
1. Can a quadrilateral have 4 obtuse angles?
No, a quadrilateral cannot have 4 obtuse angles. The sum of all interior angles in a quadrilateral is always 360 degrees. Since each obtuse angle is greater than 90 degrees, having four obtuse angles would result in a sum greater than 360 degrees, which is impossible for a quadrilateral. At most, a quadrilateral can have 3 obtuse angles.
2. Can a triangle have two obtuse angles?
No, a triangle cannot have two obtuse angles. The sum of a triangle’s interior angles is always 180 degrees, and an obtuse angle is already greater than 90 degrees. If there were two obtuse angles, their total would exceed 180 degrees, which is not possible in a triangle. Thus, a triangle can have only one obtuse angle.
3. What is the difference between an acute, right, and obtuse triangle?
- An acute triangle has all three angles measuring less than 90 degrees.
- A right triangle has one angle that is exactly 90 degrees.
- An obtuse triangle has one obtuse angle (greater than 90 degrees) and two acute angles.
4. Why did the obtuse angle go to the beach?
Because it was over 90 degrees! This joke plays on the fact that an obtuse angle is always larger than a right angle (90 degrees), making the connection between heat and geometry a little more fun!
Practice Problems
Now that you’ve learned about obtuse angles, here are some practice problems to test your understanding:
Problem 1: If an obtuse angle in a triangle measures 115 degrees, what are the measures of the other two angles?
Answer: The remaining two angles must add up to 65 degrees (180 – 115 = 65), so they could be 30 degrees and 35 degrees, for example.
Problem 2: True or False—A triangle can have an obtuse angle and a right angle.
Answer: False. The sum of an obtuse angle and a right angle would already be greater than 180 degrees, which violates the rule that the interior angles of a triangle must sum to 180 degrees.
Problem 3: If the angles of a triangle are 40 degrees, 35 degrees, and 105 degrees, what kind of triangle is it?
Answer: It is an obtuse triangle because one of the angles (105 degrees) is greater than 90 degrees.
Whether in architecture, design, or everyday objects, obtuse angles appear all around us. Understanding their properties helps deepen our knowledge of geometry and how it shapes the world. So next time you spot an angle that’s “over 90 degrees,” you’ll know exactly what it’s all about!
Interested in taking your child’s math skills to the next level? Sign up for a FREE trial class with Spark Math by Spark Education today or try our FREE Online Math Assessment for a detailed report on your child’s math skills! Spark Math is the flagship math course under Spark Education, offering small group classes taught by experienced and engaging real-life teachers. Our program is designed to ignite your child’s passion for learning math, providing a rich array of math resources and an immersive learning experience. Come and see how Spark Math can make a difference in your child’s education!




