In geometry, angles play a crucial role in understanding the relationships between different shapes and lines. One important type of angle pair is known as consecutive interior angles. These angles are commonly found when two lines are intersected by a third line, called a transversal. Understanding consecutive interior angles is essential for grasping the concepts of parallel lines and their properties. This blog will explain what consecutive interior angles are, explore relevant theorems, and provide practical examples.
What Are Consecutive Interior Angles?
Consecutive interior angles, also known as same-side interior angles, are formed when two lines are intersected by a transversal. These angles lie on the inside of the two lines and are on the same side of the transversal.
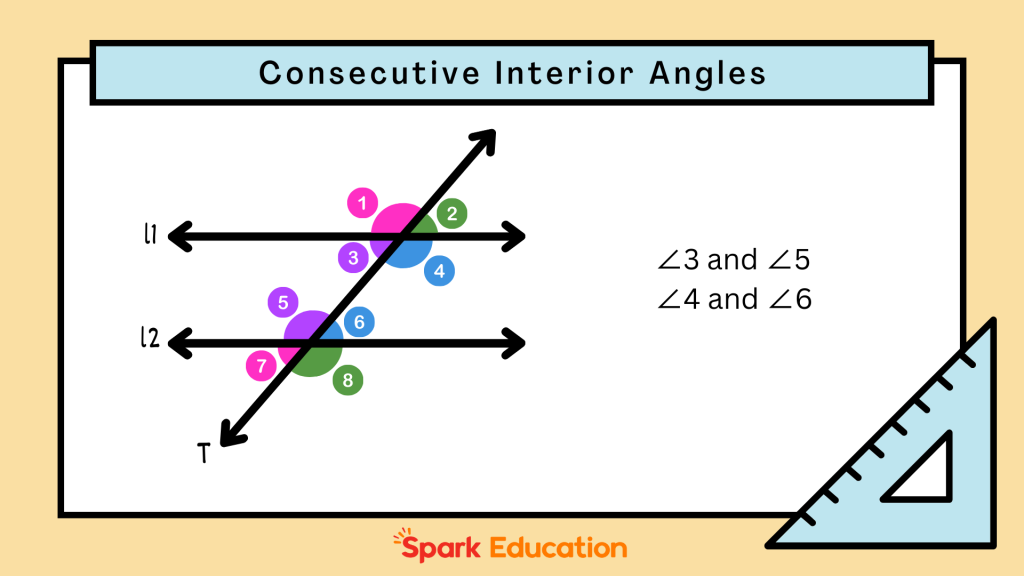
Consider two lines, Line 1 (L1) and Line 2 (L2), intersected by a transversal line (T). The intersection creates eight angles. The pairs of angles that are both inside L1 and L2 and on the same side of T are called consecutive interior angles. For instance, if we label the angles formed by the transversal as angles 1 through 8, angles 3 and 5 are consecutive interior angles, as are angles 4 and 6.
Consecutive Interior Angles Theorem
The Consecutive Interior Angles Theorem states that if two parallel lines are intersected by a transversal, then each pair of consecutive interior angles is supplementary. This means that the measures of these angles add up to 180 degrees.
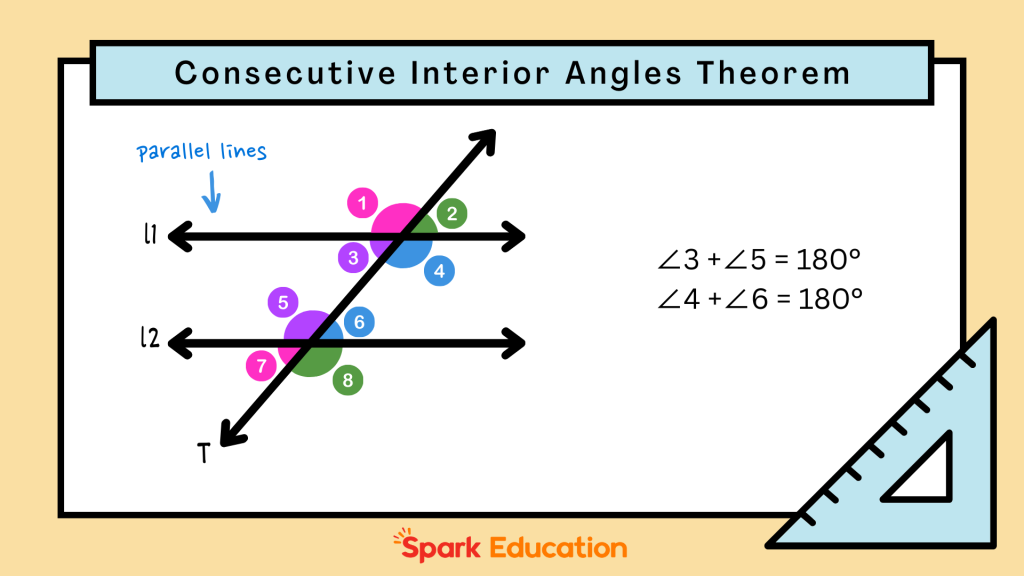
Imagine two parallel lines, L1 and L2, cut by a transversal T. The pairs of angles formed on the same side of the transversal (like angles 3 and 5, or angles 4 and 6) are consecutive interior angles. According to the theorem, if L1 and L2 are parallel, then:
∠3 + ∠5 = 180° and ∠4 + ∠6 = 180°
This property is often used in geometric proofs and problem-solving to confirm that two lines are indeed parallel or to find unknown angle measures.
Converse of the Consecutive Interior Angles Theorem
The Converse of the Consecutive Interior Angles Theorem is equally important in geometry. It states that if two lines are cut by a transversal and the consecutive interior angles are supplementary (meaning their sum is 180 degrees), then the two lines are parallel.
This theorem is often used to prove that two lines are parallel when given the angle measures. For example, if you know that ∠3 + ∠5 = 180°, you can conclude that lines L1 and L2 are parallel.
Understanding Consecutive Interior Angles in Different Scenarios
It’s important to note that consecutive interior angles can form in both parallel and non-parallel line scenarios. While their supplementary property holds true for parallel lines, it may not apply for non-parallel lines.
Parallel Lines: When the lines are parallel, consecutive interior angles are supplementary, which means their measures add up to 180°.
Non-Parallel Lines: For non-parallel lines, consecutive interior angles do not necessarily add up to 180°, and they may not exhibit any supplementary property.
Consecutive Interior Angles in a Parallelogram
In a parallelogram, pairs of adjacent angles are examples of consecutive interior angles, and they sum to 180°, just like in the parallel lines scenario.
Example: In parallelogram ABCD:
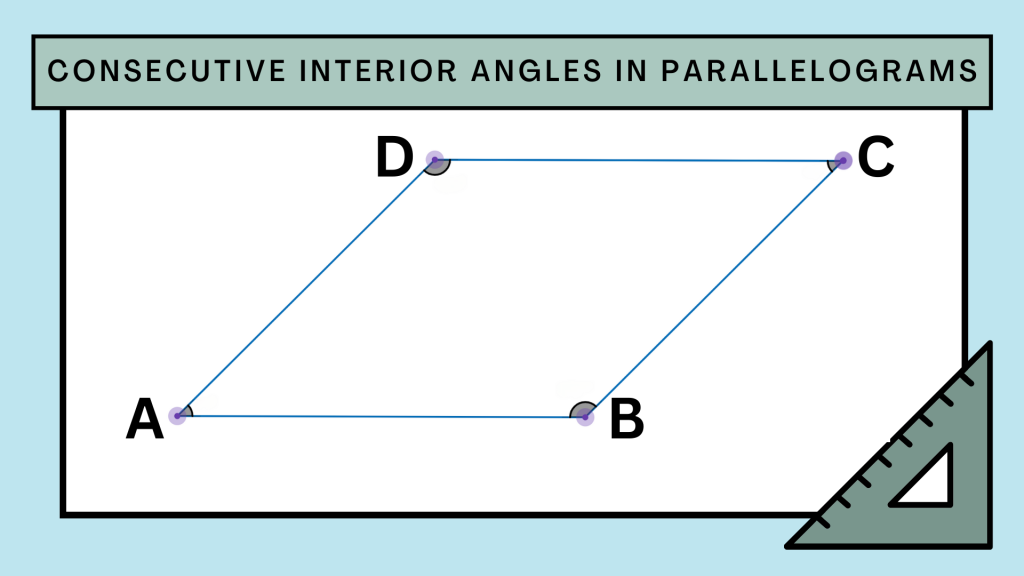
Properties:
- ∠A+∠B=180°
- ∠B+∠C=180°
- ∠C+∠D=180°
- ∠D+∠A=180°
Example Problem: Given ∠A=45°. Find ∠B.
In a parallelogram: ∠A+∠B=180°
Substitute the given value: 45∘+∠B=180°
Solving for ∠B:
∠B=135°
Common Questions and Answers
Q1. Are consecutive interior angles congruent?
One common mistake is thinking that consecutive interior angles are always congruent. While they can be congruent in specific cases (like both being 90 degrees), they are generally supplementary if the lines intersected by the transversal are parallel.
Q2. Are consecutive interior angles always supplementary?
Not necessarily. Consecutive angles are supplementary (add up to 180 degrees) only when the two lines they intersect are parallel.
Q3: What is the difference between consecutive interior and alternate interior angles?
Consecutive interior angles lie on the same side of the transversal, while alternate interior angles lie on opposite sides.
Practice Problems and Solutions
1. Find the Missing Angle
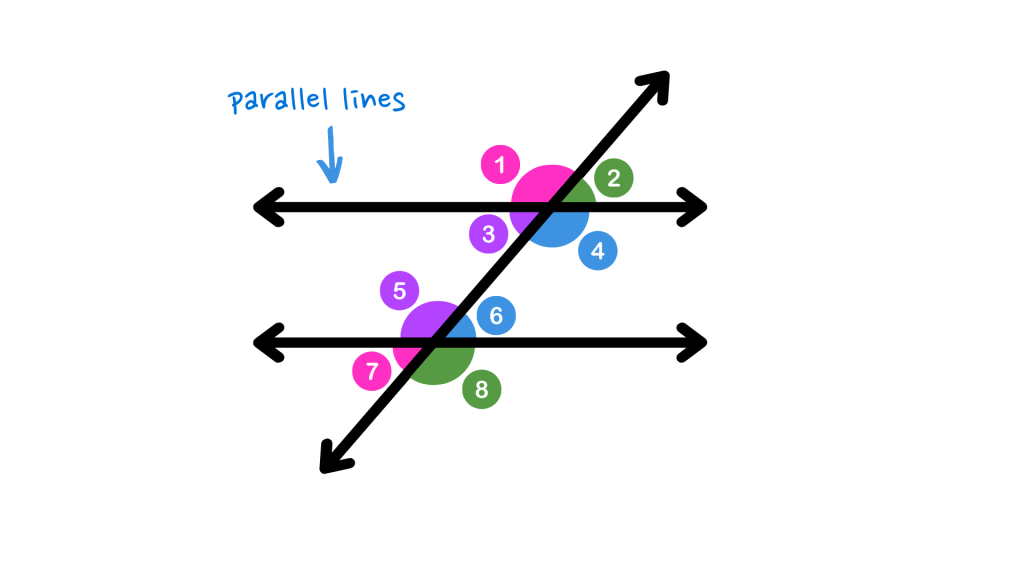
As shown in the picture below, if angle 3 measures 40 degrees, what is the measure of angle 5 (its consecutive interior angle)?
2. Prove Parallel Lines
If two lines are cut by a transversal and the consecutive interior angles measure 85 degrees and 95 degrees, are the lines parallel?
3. Find x
As shown in the picture below, find the value of x if consecutive interior angles are (3x−4)° and (x+8)°.
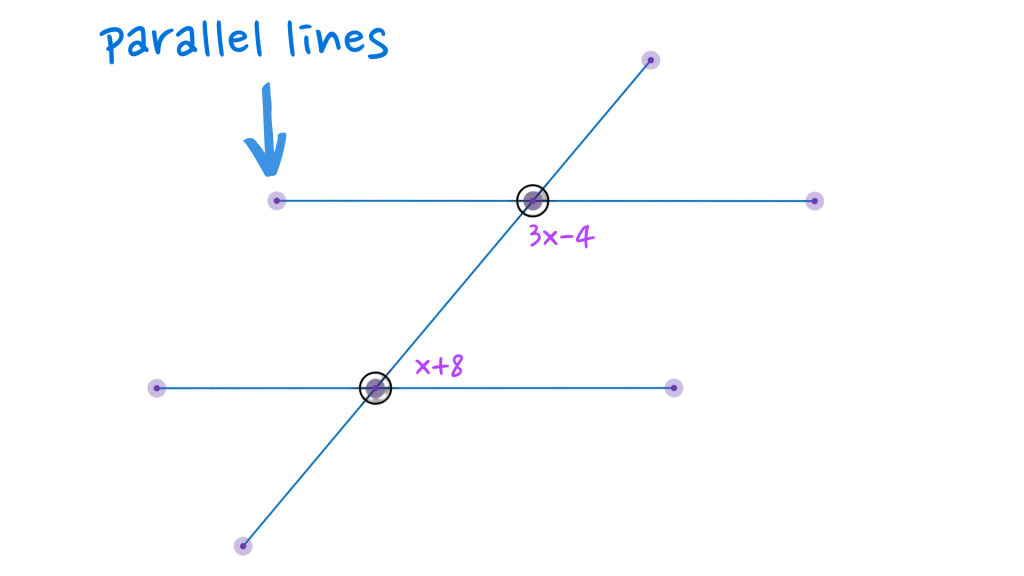
Solutions
1. Since the angles are supplementary, angle 5 would measure 140 degrees (180 – 40 = 40).
2. No, because the angles do not add up to 180 degrees, the lines are not parallel.
3. Given that the two lines are parallel, the consecutive interior angles are supplementary.
(3x − 4)° + ( x + 8)° = 180°
4x + 4 = 180
4x = 176
x = 44°
Conclusion
Consecutive interior angles are a fundamental concept in geometry that helps us understand the relationships between lines and angles. Knowing that these angles are supplementary when lines are parallel provides a valuable tool for solving geometric problems and proving the parallelism of lines. By mastering this concept, students can enhance their understanding of geometric principles and their applications in the real world.
Interested in taking your child’s math skills to the next level? Sign up for a FREE trial class with Spark Math by Spark Education today or try our FREE Online Math Assessment for a detailed report on your child’s math skills! Spark Math is the flagship math course under Spark Education, offering small group classes taught by experienced and engaging real-life teachers. Our program is designed to ignite your child’s passion for learning math, providing a rich array of math resources and an immersive learning experience. Come and see how Spark Math can make a difference in your child’s education!




