In the world of algebra, understanding the properties of equality is fundamental to solving equations and understanding mathematical relationships. These properties serve as the building blocks for manipulating equations and finding solutions. Whether you’re balancing an equation or simplifying expressions, the properties of equality ensure that whatever you do to one side of the equation, you can do to the other, keeping the equation true. This guide will introduce you to the key properties of equality, providing clear definitions, examples, and practical applications.
What are Properties of Equality?
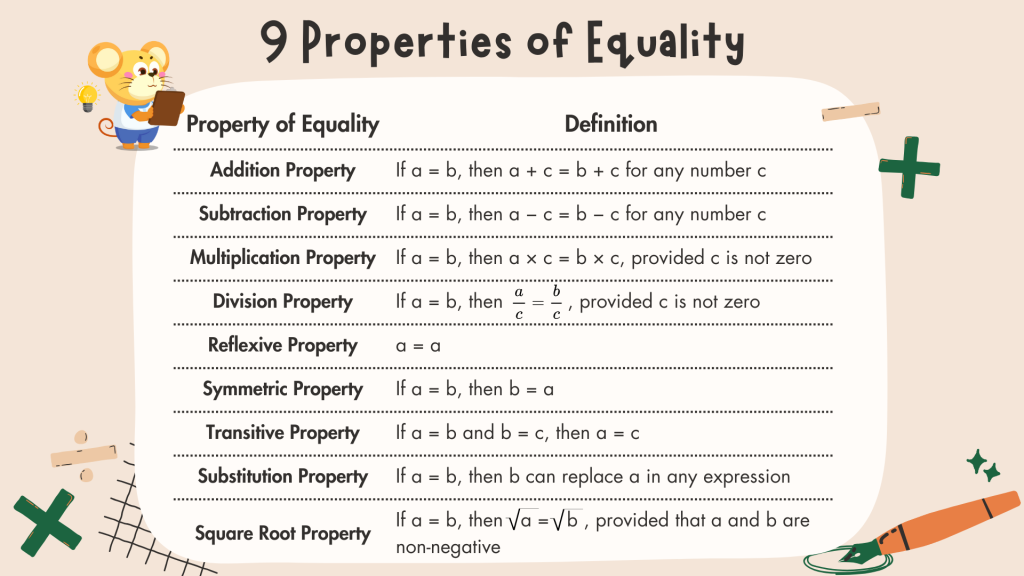
Properties of equality are fundamental mathematical rules that describe the relationship between two equal quantities. These rules ensure that when an operation is applied to one side of an equation, the same operation must be applied to the other side to maintain balance. There are nine key properties of equality: addition, subtraction, multiplication, division, reflexive, symmetric, transitive, substitution, and square root properties. The addition, subtraction, multiplication, and division properties are crucial for solving algebraic equations involving real numbers. Meanwhile, the reflexive, symmetric, and transitive properties together define equivalence relations. These properties lay the foundation for solving equations and simplifying expressions within rational, real, or complex number systems.
List of Properties of Equality
Addition Property of Equality
Definition: The addition property of equality states that if you add the same number to both sides of an equation, the equation remains balanced. In other words, if a = b, then a + c = b + c for any number c.
Example:
Consider the equation
To isolate
, add 5 to both sides:
So,
Subtraction Property of Equality
Definition: The subtraction property of equality states that if you subtract the same number from both sides of an equation, the equation remains balanced. This means that if a = b, then a − c = b − c for any number c.
Example:
Consider the equation
To isolate
, subtract 7 from both sides:
So,
Multiplication Property of Equality
Definition: The multiplication property of equality asserts that if you multiply both sides of an equation by the same non-zero number, the equation remains balanced. If a = b, then a × c = b × c, provided c is not zero.
Example:
Consider the equation
To isolate
, multiply both sides by 4:
So,
Division Property of Equality
Definition: The division property of equality states that if you divide both sides of an equation by the same non-zero number, the equation remains balanced.
That is, if a = b, then
provided c is not zero.
Example:
Consider the equation
To isolate x, divide both sides by 6:
So,
Reflexive Property of Equality
Definition: The reflexive property of equality simply states that any quantity is equal to itself. Mathematically, this is expressed as a = a.
Example: An example would be 10 = 10. This is an inherent truth in mathematics, affirming that any number is always equal to itself.
Symmetric Property of Equality
Definition: The symmetric property of equality indicates that if one value equals another, then the second value equals the first. In other words, if a = b, then b = a.
Example:
Consider if
Using the symmetric property:
This property is especially useful in proofs and algebraic manipulations.
Transitive Property of Equality
Definition: The transitive property of equality states that if one quantity equals a second quantity, and the second quantity equals a third quantity, then the first quantity equals the third. Formally, if a = b and b = c, then a = c.
Example:
If
and
then:
Substitution Property of Equality
Definition: The substitution property of equality states that if two values are equal, one can be substituted for the other in any equation or expression. If a = b, then b can replace a in any expression involving a.
Example:
Given
and the equation
substituting
with 2:
Square Root Property of Equality
Definition: The square root property of equality states that if two quantities are equal, then their square roots are also equal.
If a = b, then
provided that a and b are non-negative.
Example:
Consider the equation
To solve for
we take the square root of both sides:
so,
This shows that the square root property of equality can lead to two possible solutions (positive and negative roots).
Practical Examples
Understanding these properties is crucial, but applying them is where the real learning happens. Let’s go through a few worked problems to see these properties in action.
Example 1: Solving Equations Using Multiple Properties
Problem:
Solve for
in the equation
Solution:
Subtract 4 from both sides using the subtraction property of equality:
which simplifies to
Divide both sides by 3 using the division property of equality:
simplifying to
Example 2: Using Symmetric and Substitution Properties
Problem:
If
and
, what is
?
Solution:
By the symmetric property of equality,
since
, we also know
By substitution,
means
These examples show how these properties work together to solve equations efficiently and accurately.
Conclusion
The properties of equality are fundamental tools in algebra that ensure the integrity of equations as we manipulate them to find solutions. Whether adding, subtracting, multiplying, or dividing, these properties guarantee that the equation remains balanced and true. By mastering these properties, students can approach algebraic problems with confidence, knowing that they have the tools to maintain equality throughout their calculations.
Interested in taking your child’s math skills to the next level? Sign up for a FREE trial class with Spark Math by Spark Education today or try our FREE Online Math Assessment for a detailed report on your child’s math skills! Spark Math is the flagship math course under Spark Education, offering small group classes taught by experienced and engaging real-life teachers. Our program is designed to ignite your child’s passion for learning math, providing a rich array of math resources and an immersive learning experience. Come and see how Spark Math can make a difference in your child’s education!




