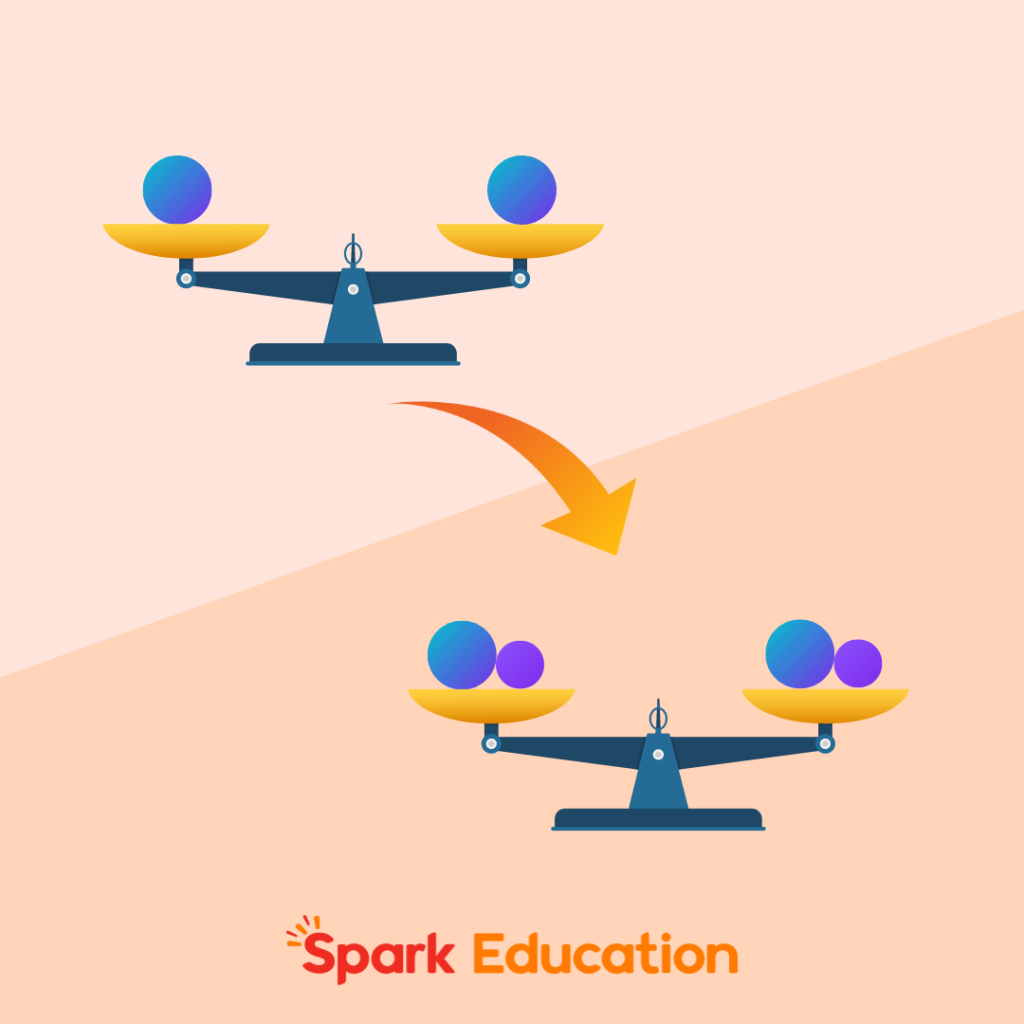
Imagine you have a set of scales perfectly balanced with equal weights on both sides. Now, what would happen if you added an identical object to each side? The scales would remain perfectly even, right? This simple concept forms the basis of the addition property of equality – a fundamental principle in mathematics that allows us to manipulate equations while preserving their balance. In this blog post, we will explore the addition property of equality in detail, providing definitions, examples, and practical applications to help you master this essential concept.
What is the Addition Property of Equality?
At its core, the addition property of equality states that when you add the same value to both sides of an equation, the equation remains balanced or equal. It’s like placing an equal number of weights on each side of a scale – the equilibrium remains undisturbed. In mathematical terms, if a = b, then a + c = b + c for any real number c.
How to Apply the Addition Property of Equality: Steps and Examples
Step-by-Step Guide
Identify the Equation: Start with an equation where you need to isolate the variable.
Determine What to Add to Both Sides: Identify the number that will help isolate the variable when added to both sides of the equation.
Simplify: Simplify both sides of the equation to solve for the variable.
Verify the Solution: Substitute the solution back into the original equation to check if it holds true.
Let’s walk through an example to see how this property works.
Example Problem
Solve the equation x − 3 = 7
Step 1: Identify the equation. Here, the equation is x − 3 = 7.
Step 2: Apply the addition property of equality. To isolate x, we need to eliminate the -3 on the left side. We do this by adding 3 to both sides of the equation:
x − 3 + 3 = 7 + 3
Step 3: Simplify both sides. On the left side, −3 + 3 cancels out, leaving x. On the right side, 7 + 3 = 10. So, the equation simplifies to:
x = 10
Step 4: Substitute x = 10 back into the original equation.
10 − 3 = 7
Since both sides are equal, the solution x = 10 is correct.
Practice Problems and Solutions
To solidify your understanding, let’s work through some practice problems.
Practice Problems:
- Solve the equation x + 9 = 14
2. Solve the equation y − 7 = 5
3. Solve the equation z + 6 = 10
4. Solve the equation x − 4 = 3
5. Solve the equation y + 2 = 11
Solutions:
- x + 9 = 14
Step: Subtract 9 from both sides.
Solution: x = 14 − 9 ⇒ x = 5
2. y − 7 = 5
Step: Add 7 to both sides.
Solution: y = 5 + 7 ⇒ y = 12
3. z + 6 = 10
Step: Subtract 6 from both sides.
Solution: z = 10 − 6 ⇒ z = 4
4. x − 4 = 3
Step: Add 4 to both sides.
Solution: x = 3 + 4 ⇒ x = 7
5. y + 2 = 11
Step: Subtract 2 from both sides.
Solution: y = 11 − 2 ⇒ y = 9
FAQs
To ensure a comprehensive understanding of the addition property of equality, let’s address some frequently asked questions:
1. Can the value added to both sides of an equation be zero?
Yes, the addition property of equality holds true even when the value added to both sides is zero. Adding zero to any number or expression does not change its value, so the equality remains intact.
2. Does the addition property of equality work for inequalities as well?
Absolutely! The addition property of equality can be extended to inequalities. If you add the same value to both sides of an inequality, the inequality relationship remains unchanged.
For example, if x > y, then x + z > y + z, where z is any real number.
3. Can negative values be added to both sides of an equation?
Certainly! The addition property of equality applies to both positive and negative values. When adding a negative value to both sides of an equation, it effectively becomes a subtraction operation.
For instance, if x + 3 = 9, then x + 3 – 3 = 9 – 3, which simplifies to x = 6.
4. How is the addition property of equality related to other properties, such as the subtraction property or the multiplication property?
The addition property of equality is closely related to other properties of equality, such as the subtraction property and the multiplication property. These properties work together to provide a comprehensive set of tools for manipulating and solving equations.
The subtraction property of equality states that if the same value is subtracted from both sides of an equation, the equality remains unchanged. The multiplication property of equality states that if both sides of an equation are multiplied by the same non-zero value, the equality is preserved. See detailed explanations in the Complete Guide to Properties of Equality in Algebra.
Conclusion
The addition property of equality is a foundational concept in algebra that plays a crucial role in solving equations. By understanding and applying this property, you can manipulate equations with confidence, knowing that you’re maintaining their balance. Whether you’re working on simple equations or real-life problems, this property will help you find solutions efficiently and accurately.
Interested in taking your child’s math skills to the next level? Sign up for a FREE trial class with Spark Math by Spark Education today or try our FREE Online Math Assessment for a detailed report on your child’s math skills! Spark Math is the flagship math course under Spark Education, offering small group classes taught by experienced and engaging real-life teachers. Our program is designed to ignite your child’s passion for learning math, providing a rich array of math resources and an immersive learning experience. Come and see how Spark Math can make a difference in your child’s education!




